Research

Shape Signature

Uniform Specifications of Shapes

Georgia Institute of Technology
2018-2019

In collaboration with:
Dr. Athanassios Economou
School of Architecture
Georgia Institute of Technology
Dr. Josephine Yu
School of Mathematics
Georgia Institute of Technology
Dr. James Park
School of Architecture
Georgia Institute of Technology
Dr. Heather Ligler
School of Architecture
Penn State University

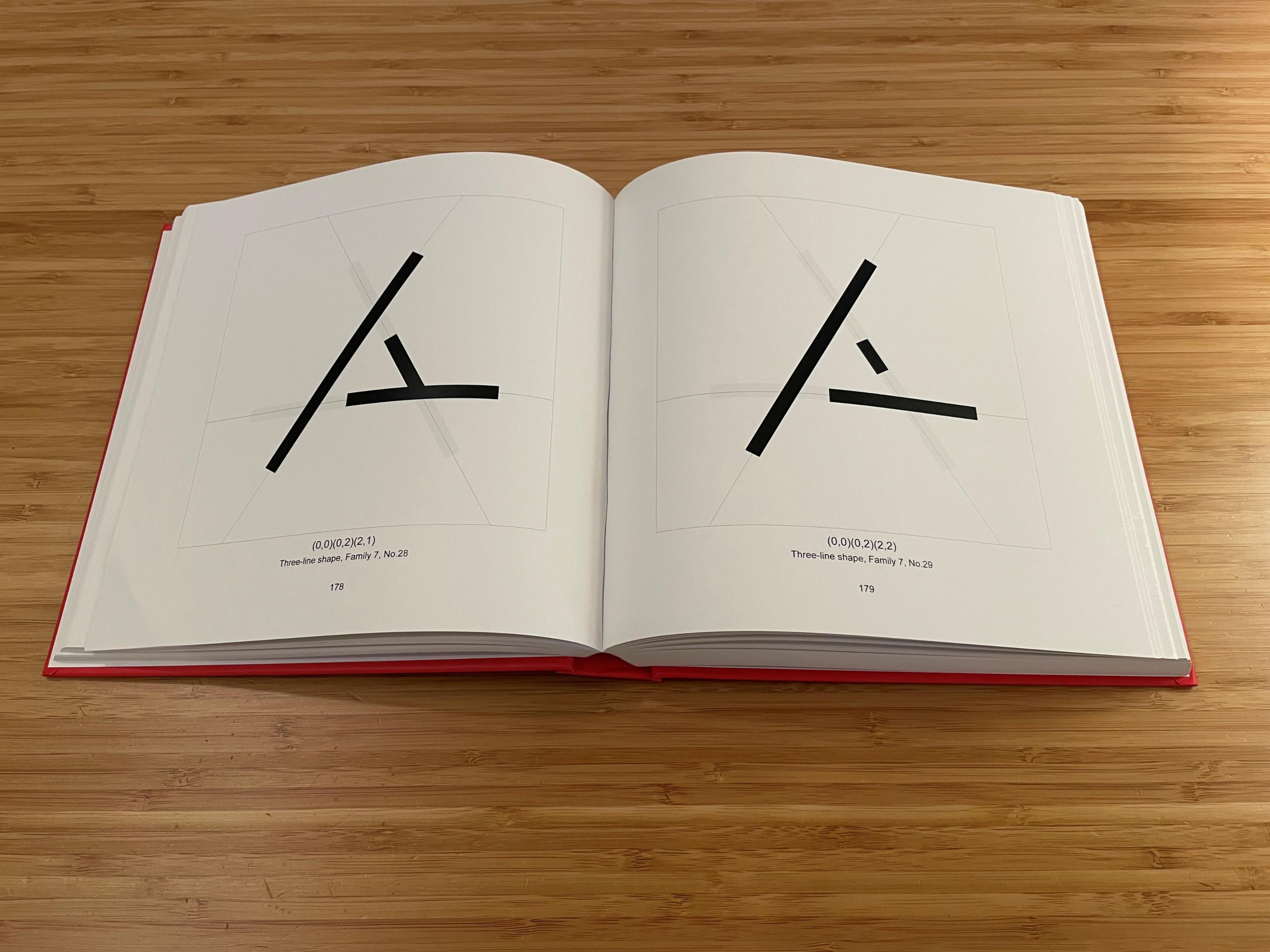
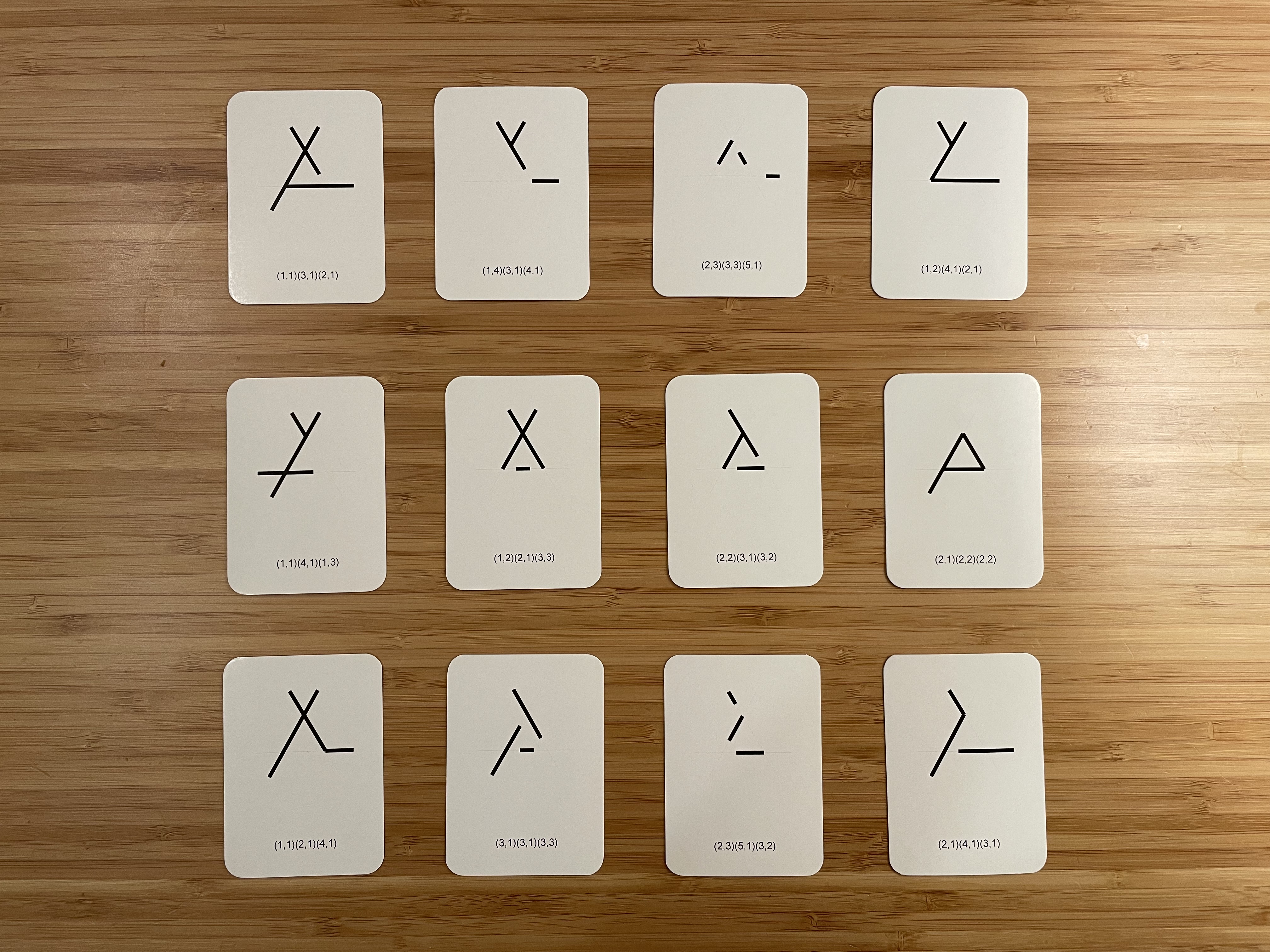
This project started from a simple question, however, that is hard to answer: How many types of shapes if we draw three straight lines on a piece of paper? If any triangle is categorized as the same type of shape, any shape “looks like” a captital ‘A’ is categorized as the same type, then, how many families we can have? This question is deeply related to design and the mathematics behind design. Similar studies such as ‘Animal of Architecture’ conducted by Dr. Lionel March can be found in the literatures of design research. We are proud to have Dr. Josephine Yu in our research group to help us derive the answer. By using Burnside Lemma and Polya technics to derive a signature (mathematical expression) of each family of shape, the result of calculation is 528 families. A key idea in the shape signature is the indefinite number of parts in a maximal element. This number is only defined with respect to the context where a maximal element is. For example, a line when it is in no spatial relation with some other line, it has 1 unlimited number of parts; the same line may have up to 5 parts when it is in a spatial relation with another line; 13 parts with 2 lines; 41 parts with 4 lines; and so forth, following the sequence of centered square figurate numbers and their corresponding powers in higher dimensions for more elaborate combinations of number of parts per line.



Demo Video on Youtube:
Shape Signature Machine